The following is a "thinking-out-loud" kind of thought experiment which sort of went off the rails... and then got righted again.
The simplicity of emptiness
We accept the simplicity of emptiness as a reliable foundation upon which to establish all of mathematics without question. The logic is plain: clearly, there can be nothing more simple than the empty set. We start there, and, knowing that we have begun with the most logically solid foundation possible, develop the rest of set theory. For a similar reason, Peano's axioms, widely understood to be fundamental, start with zero as the first number, and follow this same pattern; from simplicity to complexity.
Note that we entail a few little-considered intuitions about mathematics when we begin with emptiness in this way. For example, one of the little-realized implications of starting with emptiness is that it is then easier to accept the assumption that none of mathematics has any physical weight whatsoever. In other words, the full continuum of real numbers, in all its vast and infinitely divisible infinitude -- as well as its related dividing techniques like Dedekind cuts or Cantorian diagonals which we use to separate reals and infinities from each other -- all of this together weighs nothing.
Such weightlessness -- although a kind of emptiness -- may seem unrelated to the empty set -- another kind of emptiness, but consider: if we imagined for a moment that numbers or anything in mathematics weighed anything, a curious paradox about something resting upon nothing would immediately appear to imagination in stark relief to the elegance of the well-laid foundation.
Therefore we can be sure all of mathematics weighs nothing, even though it's not the way we normally think about math.
By means of this small thought experiment, the overlapping relationship between various forms of emptiness becomes a little more obvious; emptiness is emptiness, whether we call it zero, empty set, weightlessness, null, or any other name. It is the lack of properties which blends all these nomenclatures into one.
Much of this point is simply taken for granted. For example, no one talks about mathematics as having no weight; there is not even a need for an axiom; it is simply not questioned, being of such little consequence that it is easily ignored. "Everyone knows" mathematics has no weight.
Although emptiness is a relatively new idea within mathematics, such assumptions about the non-physical nature of mathematics are ancient; they can easily be traced back through Descartes' separation between mind and matter, to Plato's perfect ideals, or Euclid's extensionless points, and further.
A paradox at the root of set theory
Although the non-physical nature of mathematics is ancient, recent studies of the cognitive roots of mathematical concepts prove that mathematical intuition and mathematical structures originate in -- and cannot be separated from -- the same perceptual awareness we use to comprehend the physical world. There are other, similar studies, but Giuseppe Longo and Arnaud Viarouge pose a striking paradox regarding how we accept the formless empty set as the cornerstone of mathematics:
"...there is no Mathematics without structure; its constitutive analysis must be the opposite of the unstructured assembly which is the primary foundation and the conceptual origin of Set Theory." -- Mathematical Intuition and the Cognitive Roots of Mathematical Concepts
In other words, the structureless form of the empty set does not correspond with the cognitive roots of mathematical concepts. It is an artificial construction. The authors go into a fair amount of detail on this matter, saying: "In Set Theory, elements or points precede structures; the latter are conceptually secondary. In our views, gestalts, as structures, precede points, they are our primary, proto-mathematical relations to the world."
This "Foundation Paradox" is strengthened as the authors go on to discuss the remarkable invariance and high degree of certainty which are defining features of Mathematics. This is a significant point, not to be glossed over as we try to resolve the dilemma -- in fact, it points in the direction we should go with our answer: toward certainty, away from artificial structures.
This invariant nature embedded within how we understand and process mathematics can be said to shine a spotlight onto the importance of getting the foundation correct: If mathematics is the science of structures and mathematics exhibits the highest degree of invariance (indeed, the highest certainty in all of scientific inquiry) then it follows that the most stable portion of mathematics -- its foundation -- should reveal structures and parallels to origins in our perceptions and cognitions rooted in billions of years of evolutionary progress. However, we find the opposite; a complete lack of structure, an emptiness, at the root of mathematics.
How did we get here?
Infinity and zero historically separated
Without the existence of such a paradox, the novel structure proposed by this present essay would be easily disregarded, because on the surface "infinity at the beginning instead of the ends" is as absurd as claiming that anything mathematical has physical properties -- like weight, or mass, etc.
But the paradox clearly does exist. And as long as it does, we must look more closely at all possible resolutions; even those which may seem absurd at first glance.
As we shall see, the absurdity of our proposition is not an essential feature. In fact, it is more an artifact of how the history of mathematics unfolded than it is an actual mathematical impossibility.
The initial conceptual difficulty with fundamentally changing how we look at infinity is rooted in an idea which previously dominated mathematics for centuries -- but is no longer considered important, being now a footnote in the history books. This idea is known as horror vacui, or, an extremely strong prejudice against the idea of emptiness. Aristotle was the first to write of this, and his influence on this matter reached centuries into the future, until the horror began to dissipate with the arrival of the zero from India in about 600 A.D.
That dissipation was slow. For example, when the zero first came into being, nobody quite realized that it would grow from its role as a convenient, semantically meaningless, placeholder used within large numbers, into the embodiment of something substantial: the founding "nothing" or "emptiness" by which we know it today. The evolution of zero was necessarily slow, for if its emptiness as we know it had been known early, the horror would have prevented its arrival altogether.
Although avoiding emptiness, Aristotle did not avoid that which we today consider its opposite: infinity. Indeed, he had a fairly sophisticated concept of infinity, even separating infinity into two different kinds, "actual" and "potential." Thus the idea of infinity as being larger than the end of countable numbers was known and studied long before zero was placed where we have it today, at the beginning of countable numbers.
The separation in time of these two insights is important. After zero was finally accepted as a placeholder and then later as a numerical symbol for nothing, eventually negative numbers were discovered. Along with them came negative infinity, a mirror image of positive infinity. In this way, emptiness was incrementally but firmly established at the center of the wide spectrum we now know as the real number line, extending from negative infinity to positive infinity.
To summarize: The horror vacui, a fear of emptiness, was fading from its dominant place in our minds at the same time zero, or emptiness, was evolving into its now-central place within our mathematical understanding. This gradual transformation is the key to understanding why no one ever seriously considered the possibility that zero and infinity could be joined, in the manner that we will now explore.
Thus the seeming absurdity of our position is more an artifact of how the history of mathematics unfolded, than it is an actual mathematical impossibility as it appears on the surface. Now that zero and emptiness have been firmly embedded in their places within mathematics for the past century, we have the liberty to consider things which previously no one, or few, previously considered.
When infinities merge and move to the center
Let us consider, then, what happens when the two endpoints of positive and negative infinity are brought to the center of the number line, where zero firmly exists. This turns mathematics inside out. It's like starting with an ordinary 1992 Ford Taurus, dividing its engine into four pieces, and putting one fourth of the engine on each wheel.
In the process of imagining such a thing, how quickly does such an idea become incoherent? For example, can such a car even function? One answer is no, the most essential part of a car is broken. But another answer is, yes, a small motor at each wheel is how many electric cars operate. Sometimes what seems absurd can turn out to be sensible, if we give the idea a little patience.
Patience is required here. Immediately after embarking into the thought experiment about bringing two opposite infinities together, it will feel like the vastness of infinity cannot fit into the emptiness of zero. Nor can the emptiness of zero contain anything within it and still retain the essential simplicity of emptiness. On the surface, it appears one extreme will cancel the other out, either way we start. The inevitable collision feels like a zero-sum-game with only a single winner possible.
Upon further consideration however, it turns out that quantum mechanics provides a ready intuition for how to do this, by lending its concept of superposition. Superposition is where two or more separate particles inhabit the same space simultaneously. So what happens if we superpose both infinities into a single infinity, and then superpose that singularity of infinities with zero?
Before answering that, let's take a moment before going too deeply into the magical melting-pot of superposition.
Consider briefly what happens at the positive and negative ends of the number line, now that the two infinities are removed from consideration. Without infinities, both number lines simply stop when the countable numbers end. After that, nothing. Surprisingly after such a dramatic removal, the remaining structure is already well-known within mathematics, especially by computer scientists who figure out how computers should process mathematics:
By eliminating infinities from the ends of the number lines, we have not created a Frankenstein, but simply confined ourselves to computable mathematics.
Constructivists will appreciate this move immediately, as they've been saying this is the correct way for well over a century. We may quibble with finitists on the details of how this happens, but it is enough, for the moment, to realize that we need not worry too much about the ends of the number lines once we remove positive and negative infinity.
No big disaster has happened. We can set aside major structural worries for now, and safely come back to the ends later, once we've decided how to resolve what's happening in the center.
Back to superposing infinity and zero. How do we do this? Can we actually merge both infinities into a single super-infinity? Or, perhaps we place emptiness in the center of two infinities? Note, as soon as we put anything into emptiness it is no longer empty, so, should we include reference to time or do we do this instantly?
How do we get visuals on this? Beginning with what we have in the way of symbols, what happens if we place a single, merged, infinity inside the little emptiness carried visually within the number zero? That approach seems like as good a place to start as any, let's try it.
Emptiness we can imagine -- the shape of the zero comes from the shape a pebble would leave in sand after it was removed; a zero makes a good visual for emptiness -- but how do we visualize infinity? Is it like a sun, a sphere flowing outward with light? Or is it like a supernova, exploding infinitely outward, bursting all bonds, even the ability to imagine? Or somewhere between these extremes; what about a fountain... a mountain spring flowing with water into a pool...
Yes! That's it!
A mountain spring flowing outward
Imagine a mountain spring flowing outward from within a circle (say, of small stones laid around the spring in the shape of a zero, which defines the border of emptiness). The spring is overflowing the stone boundary, and a river of... numbers... are flowing outward in all directions... from emptiness?
So there's a raw visual for how to do this. It contains a paradox, of "something arriving from nothing," but we at least have a starting point. It's not too bad, as visuals go. Maybe we could use fire instead of water, an iron band instead of a few stones... but infinity is so immense, compressing it into a too-small space might cause it to explode, so let's remain with flowing water til the idea is more stable -- experiment with fires and supernovas later if the visual is moving too slow.
Is this a reasonable way to think about placing infinity within emptiness?
(Long pause).
No, not exactly. The outflowing water representing infinity has completely replaced any hope of the previously motionless, simple, emptiness that makes such a solid foundation for set theory. And all of this outward flowing is too physical. We've spent many centuries thinking of mathematics and numbers as having no physical properties and here we clearly have introduced motion, not as something studied by math, but as something mathematical; if we keep this up, we'll have to re-invent calculus, which slices physical motion into infinitesimally small, non-moving pieces. We'll need a calculus for calculus. Oh dear.
And what does a flowing number even look like?
These are problems. But then again, it's... somewhat workable. It's stable enough to hold its own while we consider some of the consequences of placing infinity at the center. It hasn't collapsed yet. It may not be perfect, but let's keep going for now.
With infinity safely flowing outward from the center, we see that the number line (going off to the right toward the greatest positive countable number, and off to the left toward the least negative countable number) can be visualized as coming out of the fountain. Little has changed for the countable integers.
The numbers start small: one, two, three, and "flow" to larger and larger... wait, larger? Larger is what happens as we get closer to infinity, and here we are going away from infinity... but getting larger...
Okay, that's a problem.
A quick fix for this is to temporarily invert the two number lines. In other words, instead of starting with the smallest countable numbers, 1,2,3,... let's imagine that the numbers flowing from the fountain at the center begin with the largest countable (whatever that is. Let's use "999" to represent it temporarily) and descend, one by one, down to a single one at the outer edge, where infinity used to be.
What happens if we do this, completing the original turn-everything-inside-out movement of infinit(ies) which began our journey? Is this even coherent?
Barely. It's getting awkward... but it's still possible to imagine, even to draw a simple line-drawing representation to help visualize what is being described here:
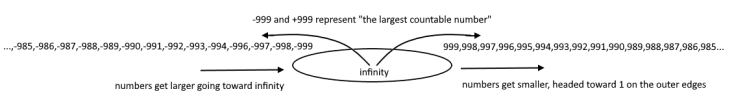
Infinity is at the center, and "the largest countable number" is nearest it, with the counting descending as the number line goes outward. This means that 1 and -1 are at the outermost edges. Since we know we're working in the computable realm, let's give realistic boundaries and say that "the largest countable number" is at the limit of whatever memory space we have available within a given computer.
Is there anything useful with this visualization, or did that last number-line-inversion cause it to lose all coherence? Can we even do something as basic as counting with this new inside-out structure? What do we do with 1 and -1 dangling in their vulnerable smallness at the outer edges?
Maybe we should undo that last temporary inversion and try another path?
Lastly, is there is anything in Nature that looks like this structure, maybe something we can use as an analogy to help think about things? Maybe a solar system, with a sun at the center, and smaller planets off toward the outer edges?
(That was an even longer pause.)
Okay, so a few days have passed, and I've been thinking about this often, trying to solve these riddles that I've created for myself. I've almost given up on the ridiculousness of where I've gotten myself in this thought experiment several times, but intuition keeps pulling me back to the image that I created above and finally, moments ago (early a.m. May 3, 2021) I just realized yes, there is something in Nature that works very much like what I just described in the illustration above!
The solution is in, quite literally, the last place I'd normally ever look: my own imagination!
Is imagination like a fountain of information out of nowhere?
Look at that illustration above and you should be able to see that it is an accurate structural representation of what your own imagination does when it is counting.
We tend to think that counting is a linear thing, but take a few minutes to think about what happens when you count. Is it linear? When you iterate a new number at the end of all that you've counted, say, as you have carefully, one-by-one, reached the number 999, and you're about to count the next number, 1,000. Where does that new number come from? Does it not come from within the center of your imagination, or, out of nowhere out of "an empty set" and suddenly it exists, matching all the patterned rules that define what number it should be (i.e. Peano's axioms). A new number is effectively a new creation, pushing all the numbers that have come before it out to the edges, like water flowing from a spring.
Now that is a dumbstrucking realization.
I've never seen the structure of my own thoughts in that way before. This is a big enough insight that I'm ready to draw this little essay to a conclusion and consider it complete enough for now, because it turned up something potentially useful.
As a conclusion, this is not where I was headed, but... I did find something coherent and insightful right when I thought I had created a mess, and this gem, of understanding what counting looks like -- what it really looks like -- is absolutely worth the journey.
Also, I have the rather pleasant confirmation that intuition was correct in drawing me back to this paradox over and over, not giving up, until it got solved. Good job, intuition.
Now I'm pretty certain I can take the ideas I was writing in the first half of this essay and drive them to a better conclusion.
Good Lord, this adventure (over the past few months contemplating these kinds of ideas and continuing to find gems like this) is truly off the rails and I can't wait to see what happens next.